Hertz, cent and decibel
These three concepts can be considered the standard measuring units of music acoustics.
Hz (hertz)
Hertz is the standard measure of the frequency of oscillations in a wave motion. The frequency is 1 Hz when one oscillation occurs in one second. When 1000 oscillations occur in one second, the frequency is 1000 Hz, or 1 kHz (kilohertz).
Pitch names start from the C of the subcontraoctave (C2). The frequency of the C2 is approximately 16 Hz (oscillations per second). This frequency is virtually impossible to perceive. In instruments such as the pipe organ it is augmented with a variable number of partial tones that enable the listener to detect the musical pitch of the tone.
When the frequency is in the range of several thousands Hz, the sound can still be heard, but defining its exact pitch becomes problematic. Pitches beyond the six-lined C (C6, 8370 Hz) are usually not defined. It is very difficult to determine the pitch of tones any higher than this.
As mentioned earlier, frequencies can be detected up to approximately 20 kilohertz (kHz). This limit is, however, more or less approximate and depends of a variety of factors, such as the intensity of the sound. Young individuals are able to detect even higher sounds (as something more than mere pressure variations occurring inside the ear). The upper limit of the hearing range, however, unavoidably falls with age, and many elderly individuals may have trouble hearing even loud sounds below 10 kHz.
Defining the hearing range as the range between 16 Hz and 20 kHz (or any other range) cannot therefore be considered more than a highly artificial average that only pertains to young population on the whole.
The following equations hold true between frequency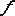 
, wavelength 
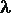and sound velocity 
:
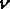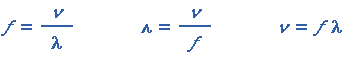
If the frequency of a sound is 440 Hz (the tone A1) and the velocity of the sound is approximately 330 m/s, its wavelength is 0.75 metres (or 75 centimetres). The successive compressions of the sound wave are 75 centimetres apart.
Sound velocity depends on medium (such as metal, water or air) and temperature. The velocity of sound propagating through air is 331.4 m/s at a temperature of 00C, but increases at a rate of 0.6 m/s per degree. The velocity of sound at room temperature (220C) is approximately 345 m/s. In the following exercise, you will have an opportunity of seeing for yourself how sound velocity is affected when the air temperature changes.
What is the speed of sound in a Finnish sauna (+80oC)?
What is the speed of sound on a cold winter day (-30oC)?
Sounds higher than 20 kHz are called ultrasounds, while sounds below 16 Hz are called infrasounds. The name of the unit, hertz, refers to the German physicist Rudolf Hertz (18571894), who first proved the propagation of radio waves empirically.
C (cent, from Lat. centum one hundred)
The unit most commonly used to measure microintervals is called cent (C; German Cent, Latin centum, meaning one hundred). It stands for one hundredth of an equal-tempered semitone. In other words, one octave consists of 1200 cents.
An equal-tempered whole tone can be expressed as 200 C, while an equal-tempered fifth would be 700 C, or seven semitones.
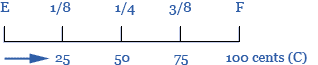
Cents are used to express the size of individual microintervals, but also to illustrate the ratio of any given interval (such as a second or a third). The differences between the intervals of different tuning systems can be compared easily when the intervals are expressed as cents. In an equal-tempered system the intervals are always multiples of one hundred. The figure below shows a comparison of typical fraction notations of microintervals and the corresponding values in cents between the tones E and F. As the figure illustrates, a quarter tone is 50 C, while an eighth tone is 25 C.
Fractions have traditionally also been used to express interval ratios. The use of fractions has been natural because the Pythagorean tuning system and the tuning system called just intonation are based on them. Comparing the fractions is, however, often more difficult than using the corresponding decimal values. It may be difficult to discern that 16/9, for example, is actually greater than 7/4 (1.78 > 1.75).
Fractions expressing interval ratios can be converted to cents using the following conversion formula:
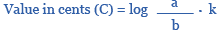
The symbols a and b correspond to the two parts of the fraction, while k is the constant 1200/10log 2 = 3986,313715...
A pure fifth, for example, is usually expressed as 3/2. The logarithm of this value is approximately 0.176.
When multiplied by k, the value is approximately 702. In other words the pure fifth is two cents wider than an equally tempered fifth. The pitch of the A3, for example, is 220 Hz. An E4, therefore, is approximately 330 Hz (3/2 · 220). An A4 is 440 Hz, while an E5 is approximately 660 Hz (3/2 * 440). A given interval will be very different in the lowest or the highest part of the hearing range. The range between 40 and 80 Hz and the range between 4000 and 8000 Hz both constitute an octave. The same interval can be expressed as a ratio in two different ways, but the higher figure is always the high tone. If, for example, the ratio of a fifth is expressed as 3/2 instead of 2/3, the cent value becomes negative (one fifth down, or -702).
The cent value can be converted to a decimal value by dividing it with the constant k and then inserting the result as the exponent of 10. Example: 702 C/k = 0.176 and 10 0,176 = 1.5, or 3/2.
Hertz values can be converted to cents and vice versa by entering the values in the calculator below. Please use a point as the decimal separator.
|
db (decibel)
Decibel is the proportional unit of sound volume. The lowest sound audible with a normal (average) hearing is 0 dB (2 ∙ 10-5 pascals as a sound pressure value or 10-12 W/m2 as an intensity value at 1000 Hz). A person with good hearing can hear even weaker sounds (-5 dB). When the intensity of the sound (the amount of energy flowing across a surface) doubles, the proportional volume of the sound increases by 3 decibels. When the sound pressure doubles, the intensity quadruples, meaning an increase of 6 dB. A hundredfold increase of sound power represents a tenfold increase of sound pressure, but only an increase of 20 dB in decibels. The decibel scale is logarithmic.
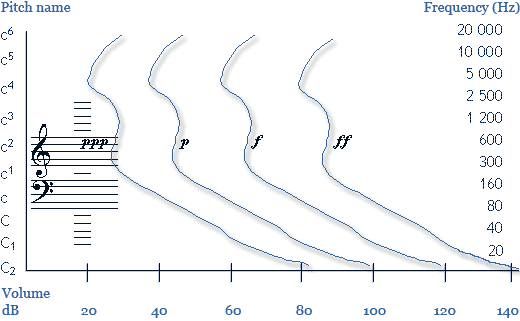
The perceived volume of a sound depends on the level of sound pressure and the pitch of the sound. Certain frequencies are more easy to hear than others. Sometimes the perceived sound volume is referred to as reception. The figure below portrays the hearing range in musical notation using tone names on the left and hertz values on the right. As the image illustrates, the most sensitive hearing range of the ear is situated approximately around the four-line octave. The level of sound pressure is measured today using a weighted decibel scale (such as dbA) that takes into account the fact that the human ear is not a measuring instrument but has different sensitivities for different frequencies.
The concepts of phon and sone are used for measuring the loudness of an auditory sensation. When using phons, the perceived sound is compared to a reference frequency of 1000 Hz. When the sounds are perceived as equally strong, they are assigned the same value, although the actual sound pressure levels might differ considerably. The sone value of a sound is also determined using a comparison: when a sound is perceived as two times as strong as the sound originally heard, it is given a double sone value. The units are subjective and very difficult to measure.
The piano and forte curves of the figure are also highly approximate. They do, however, illustrate that the low C1 (32 Hz), for example, is perceived as piano pianissimo, while a C5 (approximately 4200 Hz) with an equal decibel value is perceived as forte.
