Suomeksi | In English

Sine wave graphs

y = sin x

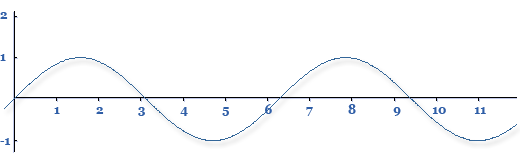
Siniaalto

This waveform illustrates the fundamental tone (a sound that includes only one frequency, a sine wave).

y = sin x + (1/2) sin 2x

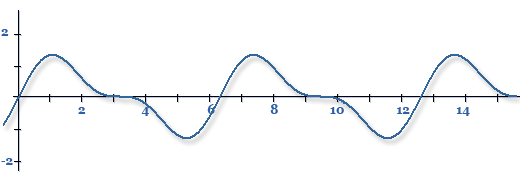
Toinen aalto

This waveform is the sum curve of the fundamental tone and its second harmonic (i.e. an octave).

y = sin x + (1/2) sin 2x + (1/3) sin 3x + (1/4) sin 4x + (1/5) sin 5x + (1/6) sin 6x

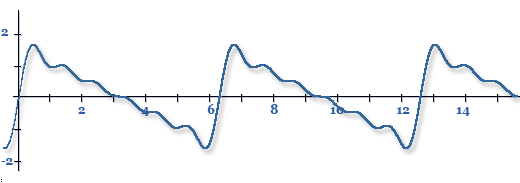
Kolmas aalto

This waveform is the sum curve of the first six harmonics.
It is starting to resemble the sawtooth wave that results when the waveform features a fundamental tone and all of its multiples (harmonics), each 6 dB weaker than the previous harmonic.

y = sin x + (1/3) sin 3x + (1/5) sin 5x + (1/7) sin 7x + (1/9) sin 9x

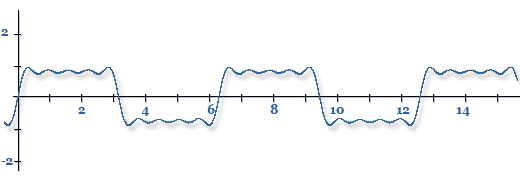
Neljäs aalto

This waveform is the sum curve of the first five odd harmonics. It resembles the square wave, a waveform that contains all the odd harmonics. Successive harmonics are, again, weaker than each previous harmonic.

Self-study exercise: Wave forms

Use the applet below to examine how different waveforms that can be used for producing different timbres electronically look and sound like. The square wave and the triangular wave only contain odd harmonics in varying ratios, while the sawtooth wave and the pulse wave include all harmonics. Volume can be adjusted using the push button. The decibel value 0 is the highest volume available. Attenuation is expressed as negative decibel values. The difference between the lowest and the highest sound is 60 dB.

Waveform experiments with the JSyn plug-in (part 1).
Waveform experiments with the JSyn plug-in (part 2).